Question 1:
The time taken, t in hour, to mow a lawn varies directly as the area of a field, L in m2, and inversely as the number of workers, w. Given that 5 workers need 3 hours to mow the grasses of the field with area of 4 × 104 m2.
(a) Find the equation that relate t in terms of L and w. [2 marks]
(b) Calculate the minimum number of workers needed to mow the grass of a field with area of 1.6 × 105 m2 within 8 hours. [2 marks]
Solution:
(a)
t∝Lt∝1wt∝Lwt=kLwt∝Lt∝1wt∝Lwt=kLw
(b)
Given that w=5,t=3,L=4×1043=k(4×104)5k=154×104=38000 Thus, t=3L8000w Given that w=5,t=3,L=4×1043=k(4×104)5k=154×104=38000 Thus, t=3L8000w
When t=8,L=1.6×1058=3(1.6×105)8000ww=3(1.6×105)8000×8=7.5=8 workers (minimum) When t=8,L=1.6×1058=3(1.6×105)8000ww=3(1.6×105)8000×8=7.5=8 workers (minimum)
The time taken, t in hour, to mow a lawn varies directly as the area of a field, L in m2, and inversely as the number of workers, w. Given that 5 workers need 3 hours to mow the grasses of the field with area of 4 × 104 m2.
(a) Find the equation that relate t in terms of L and w. [2 marks]
(b) Calculate the minimum number of workers needed to mow the grass of a field with area of 1.6 × 105 m2 within 8 hours. [2 marks]
Solution:
(a)
t∝Lt∝1wt∝Lwt=kLwt∝Lt∝1wt∝Lwt=kLw
(b)
Given that w=5,t=3,L=4×1043=k(4×104)5k=154×104=38000 Thus, t=3L8000w Given that w=5,t=3,L=4×1043=k(4×104)5k=154×104=38000 Thus, t=3L8000w
When t=8,L=1.6×1058=3(1.6×105)8000ww=3(1.6×105)8000×8=7.5=8 workers (minimum) When t=8,L=1.6×1058=3(1.6×105)8000ww=3(1.6×105)8000×8=7.5=8 workers (minimum)
Question 2:
Diagram 1 in the answer space shows a square PQRS drawn on a square grid with sides of 1 unit. Y is a point that move inside the square.
(a) On Diagram 1, draw the locus of point Y which always moves 3 units from point K. [1 mark]
(b) Calculate the distance travelled by locus Y in 2(a). [2 marks]
Solution:
(a)
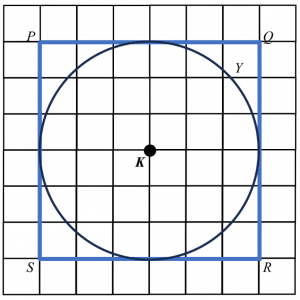
(b)
Distance travelled by locus Y (Circumference of circle) =2×π×r=2×227×3=1327=1867units
Diagram 1 in the answer space shows a square PQRS drawn on a square grid with sides of 1 unit. Y is a point that move inside the square.
(a) On Diagram 1, draw the locus of point Y which always moves 3 units from point K. [1 mark]

(b) Calculate the distance travelled by locus Y in 2(a). [2 marks]
Solution:
(a)

(b)
Distance travelled by locus Y (Circumference of circle) =2×π×r=2×227×3=1327=1867units