Question 3:
Malware Internet company has 5 Internet channels labelled P, Q, R and S to send messages.

Draw a graph with multiple edges and loops based on the given information. Hence, determine the sum of degrees.
[3 marks]
Solution:
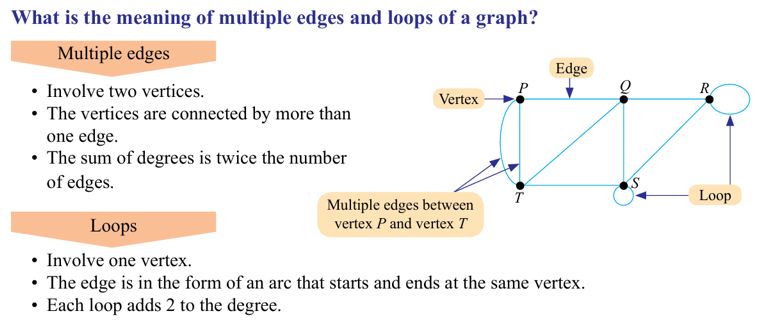
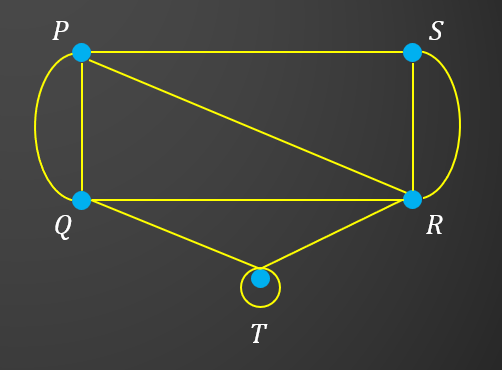
Sum of degrees =2× Number of edges =2×10=20
Malware Internet company has 5 Internet channels labelled P, Q, R and S to send messages.
Table 1 shows the number of messages that can be sent by each channel.

Draw a graph with multiple edges and loops based on the given information. Hence, determine the sum of degrees.
[3 marks]
Solution:


Sum of degrees =2× Number of edges =2×10=20
Question 4:
(a) State the antecedent and consequent for the following statement. [2 marks]
If x is a multiple of 6 , then x is a multiple of 3.
(b) Write the converse for the following implication. Hence, determine whether the converse is true or false.
[2 marks]
If x=30∘, then sinx=0.5
Solution:
Implication “If p , then q “
A statement “if p, then q” is known as an implication where
– p is denoted as the antecedent.
– q is denoted as the consequent.
(a)
Antecedent : x is multiple of 6
Consequent: x is multiple of 3
(b)
Converse ∼ If sinx=0.5, then x=30∘
False, because if sinx=0.5,x consists of two values (1st and 2nd quadrant),
x=30∘ or x=(180∘−30∘)=150∘
(a) State the antecedent and consequent for the following statement. [2 marks]
If x is a multiple of 6 , then x is a multiple of 3.
(b) Write the converse for the following implication. Hence, determine whether the converse is true or false.
[2 marks]
If x=30∘, then sinx=0.5
Solution:
Implication “If p , then q “
A statement “if p, then q” is known as an implication where
– p is denoted as the antecedent.
– q is denoted as the consequent.
(a)
Antecedent : x is multiple of 6
Consequent: x is multiple of 3
(b)

Converse ∼ If sinx=0.5, then x=30∘
False, because if sinx=0.5,x consists of two values (1st and 2nd quadrant),
x=30∘ or x=(180∘−30∘)=150∘