Section A
40 marks
Answer all questions
Question 1 (4 marks):
Diagram 1 shows a Cartesian plane.
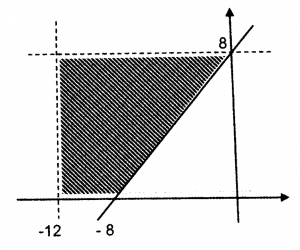 Diagram 1
Diagram 1
Write four inequalities that define the shaded region.
Answer:
(i)
(ii)
(iii)
(iv)
Solution:

(i)
For y = 8
The inequality of the shaded region below the dashed line is y < 8.
(ii)
For x = -12
The inequality of the shaded region on the right of the dashed line is x > -12.
(iii)
For y = 0
The inequality of the shaded region above the x-axis is y ≥ 0.
(iv)
y=mx+cc=8m=−8−8=1∴y=x+8
The inequality of the shaded region above the solid line is y ≥ x + 8.
40 marks
Answer all questions
Question 1 (4 marks):
Diagram 1 shows a Cartesian plane.
 Diagram 1
Diagram 1Write four inequalities that define the shaded region.
Answer:
(i)
(ii)
(iii)
(iv)
Solution:

(i)
For y = 8
The inequality of the shaded region below the dashed line is y < 8.
(ii)
For x = -12
The inequality of the shaded region on the right of the dashed line is x > -12.
(iii)
For y = 0
The inequality of the shaded region above the x-axis is y ≥ 0.
(iv)
y=mx+cc=8m=−8−8=1∴y=x+8
The inequality of the shaded region above the solid line is y ≥ x + 8.
Question 2 (4 marks):
Given the area of a triangle, A, varies directly with the constant, k, with height, x cm and base, y cm. Rajoo has a triangular-shaped piece of cardboard with x and y are 80 cm and 48 cm respectively. Calculate the value of k if the area of the cardboard is 15 360 cm². Next calculate the value of A if Rajoo has another piece of cardboard with the sum of x and y is 180 cm where the ratio of x to y is 5 : 4.
Solution:
A α xy
A = kxy
A = 15 360, x = 80, y = 48
15 360 = k × 80 × 48
15 360 = 3 840 k
k = 4
x + y = 180
x : y = 5 : 4
x=59×180 =100y=49×180 =80∴A= 4×100×80 = 32 000
Given the area of a triangle, A, varies directly with the constant, k, with height, x cm and base, y cm. Rajoo has a triangular-shaped piece of cardboard with x and y are 80 cm and 48 cm respectively. Calculate the value of k if the area of the cardboard is 15 360 cm². Next calculate the value of A if Rajoo has another piece of cardboard with the sum of x and y is 180 cm where the ratio of x to y is 5 : 4.
Solution:
A α xy
A = kxy
A = 15 360, x = 80, y = 48
15 360 = k × 80 × 48
15 360 = 3 840 k
k = 4
x + y = 180
x : y = 5 : 4
x=59×180 =100y=49×180 =80∴A= 4×100×80 = 32 000