Question 15:
(a) It is given that [7−2x+34]=[75y−14].
(ii) Diagram 9 shows the discounts given by the supermarket on a particular day.
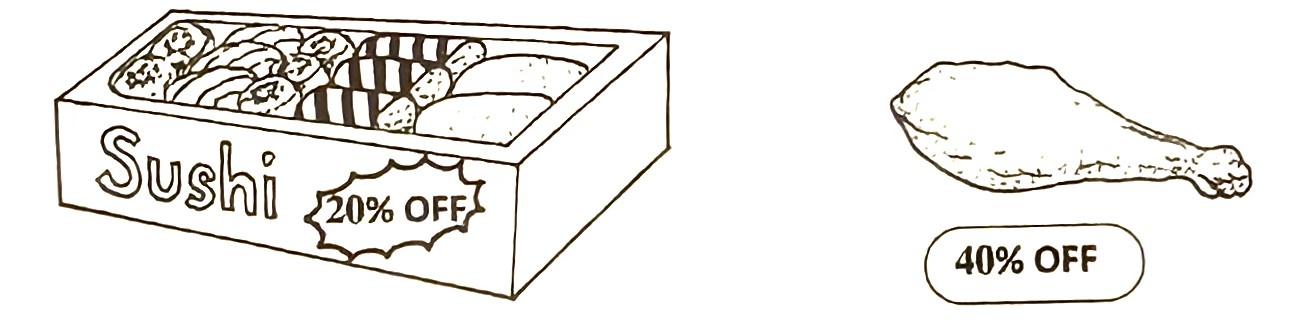
Jane has RM50. She wants to buy 2 boxes of sushi and 8 pieces of fried chicken. Does she have enough money to buy these items at the discounted price? Justify your answer by using matrix multiplication. [3 marks]
Solution:
(a)
[7−2x+34]=[75y−14]x+3=−1x=−45y=−2y=−25
(b)(i)
3x+2y=55∼ (Lily) 4x+3y=55+20∼ (Kelly) 4x+3y=75
[3243][xy]=[5575][xy]=1(3)(3)−(2)(4)[3−2−43][5575][xy]=[(3×55)+(−2×75)(−4×55)+(3×75)][xy]=[165−150−220+225][xy]=[155] A box of sushi = RM15 A piece of fried chicken = RM5
(b)(ii)
A box of sushi at discounted price =15×80100= RM12
A piece of fried chicken at discounted price =5×60100= RM3
2 boxes of sushi and 8 pieces of fried chicken in matrix multiplication =[28][123]=[2×12+8×3]=[48]
Therefore, Jane has enough money as only RM48 is needed.
(a) It is given that [7−2x+34]=[75y−14].
Determine the value of x and of y. [2 marks]
(b) A supermarket sells boxes of sushi and pieces of fried chicken.
(i) Lily spent RM55 on 3 boxes of sushi and 2 pieces of fried chicken. Kelly spent RM20 more compared to Lily on 4 boxes of sushi and 3 pieces of fried chicken. Using matrix method, calculate the price of a box of sushi and of a piece of fried chicken. [5 marks]
(ii) Diagram 9 shows the discounts given by the supermarket on a particular day.

Jane has RM50. She wants to buy 2 boxes of sushi and 8 pieces of fried chicken. Does she have enough money to buy these items at the discounted price? Justify your answer by using matrix multiplication. [3 marks]
Solution:
(a)
[7−2x+34]=[75y−14]x+3=−1x=−45y=−2y=−25
(b)(i)
3x+2y=55∼ (Lily) 4x+3y=55+20∼ (Kelly) 4x+3y=75
[3243][xy]=[5575][xy]=1(3)(3)−(2)(4)[3−2−43][5575][xy]=[(3×55)+(−2×75)(−4×55)+(3×75)][xy]=[165−150−220+225][xy]=[155] A box of sushi = RM15 A piece of fried chicken = RM5
(b)(ii)
A box of sushi at discounted price =15×80100= RM12
A piece of fried chicken at discounted price =5×60100= RM3
2 boxes of sushi and 8 pieces of fried chicken in matrix multiplication =[28][123]=[2×12+8×3]=[48]
Therefore, Jane has enough money as only RM48 is needed.