Question 7:
(a) Diagram 4 shows the incomplete steps in financial management process.

(b) Table 2 shows the income and expenses of Amelia and Julia in month of January.
Solution:
(a) R = Reviewing and revising the progress
(b)
Income = net salary, sales commission, rent received
Expenses = housing loan, utility bills, Insurance premium
Financial position of Amelia =RM(3500+400+800)−RM(1300+650+200)= RM4 700+RM2150= RM2 550
Financial position of Julia = RM (3000+150+850)−RM(1800+700+300)= RM4 000+ RM2 800= RM1 200
(a) Diagram 4 shows the incomplete steps in financial management process.

State the step labelled R. [1 mark]
(b) Table 2 shows the income and expenses of Amelia and Julia in month of January.

Between Amelia and Julia, who has the best financial position? Justify your answer. [3 marks]
(a) R = Reviewing and revising the progress
(b)
Income = net salary, sales commission, rent received
Expenses = housing loan, utility bills, Insurance premium
Financial position of Amelia =RM(3500+400+800)−RM(1300+650+200)= RM4 700+RM2150= RM2 550
Financial position of Julia = RM (3000+150+850)−RM(1800+700+300)= RM4 000+ RM2 800= RM1 200
Amelia has better financial position because surplus of income of Amelia is more than Julia.
Question 8:
Diagram 5 shows two ropes AE and BD tied in a parallel way to the vertical wooden pole EC.
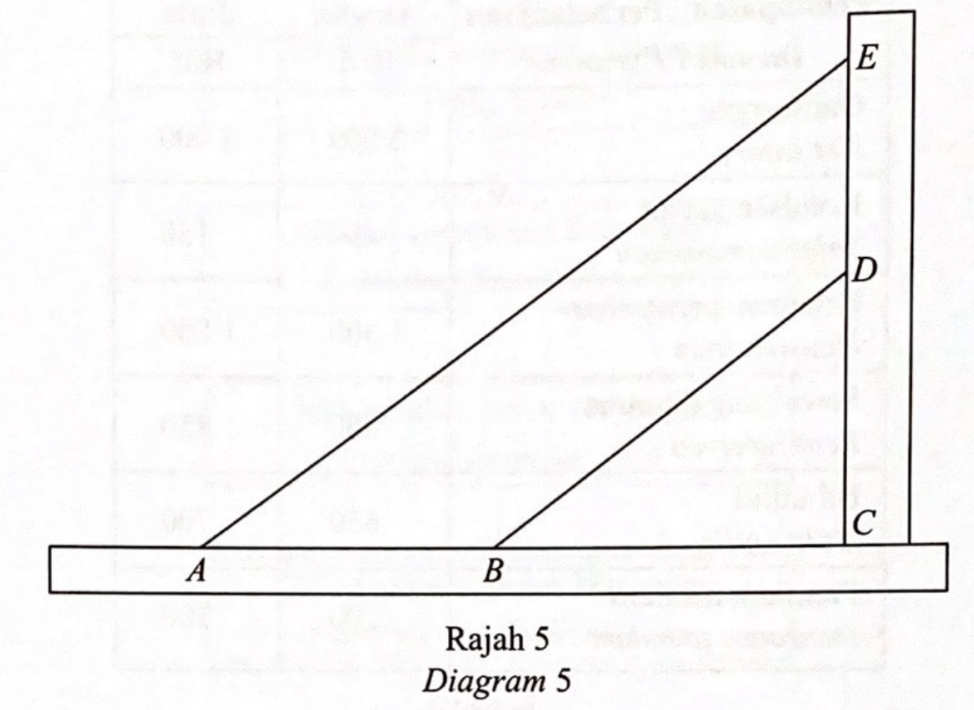 Given the equation of straight line BD is 4y – 3x = 48 and the ratio of distance AB : BC is 3 : 4.
Given the equation of straight line BD is 4y – 3x = 48 and the ratio of distance AB : BC is 3 : 4.
Find,
(a) the equation of the straight line AE, [3 marks]
(b) the distance of ED. [1 mark]
Solution:
(a)
At point E, y coordinate =04(0)−3x=48x=−483x=−16
Given that ABBC=34AB16=34AB=16×34AB=12
Thus, point A=(−16−12),0)=(−28,0)
4y−3x=48y=34x+484y=34x+12m=34
mAE=mBD=34y=34x+c,(−28,0)0=34(−28)+cc=21
Equation of the straight line AE, y=34x+21
(b)
y-intercept of the equation, y=34x+12 is 12
Thus, point D=(0,12)
y-intercept of the equation, y=34x+21 is 21
Thus, point E=(0,21)
Distance of ED=21−12=9 units
Diagram 5 shows two ropes AE and BD tied in a parallel way to the vertical wooden pole EC.
 Given the equation of straight line BD is 4y – 3x = 48 and the ratio of distance AB : BC is 3 : 4.
Given the equation of straight line BD is 4y – 3x = 48 and the ratio of distance AB : BC is 3 : 4.Find,
(a) the equation of the straight line AE, [3 marks]
(b) the distance of ED. [1 mark]
Solution:
(a)
At point E, y coordinate =04(0)−3x=48x=−483x=−16
Given that ABBC=34AB16=34AB=16×34AB=12
Thus, point A=(−16−12),0)=(−28,0)
4y−3x=48y=34x+484y=34x+12m=34
mAE=mBD=34y=34x+c,(−28,0)0=34(−28)+cc=21
Equation of the straight line AE, y=34x+21
(b)
y-intercept of the equation, y=34x+12 is 12
Thus, point D=(0,12)
y-intercept of the equation, y=34x+21 is 21
Thus, point E=(0,21)
Distance of ED=21−12=9 units