Question 13:
A Selangor state-level STEM quiz competition was conducted to select a representative to the national level. Two participants were involved in the final selection, namely Faris and Danesh. The stem-andleaf plot in Diagram 8 shows the scores obtained by the two participants after several rounds.
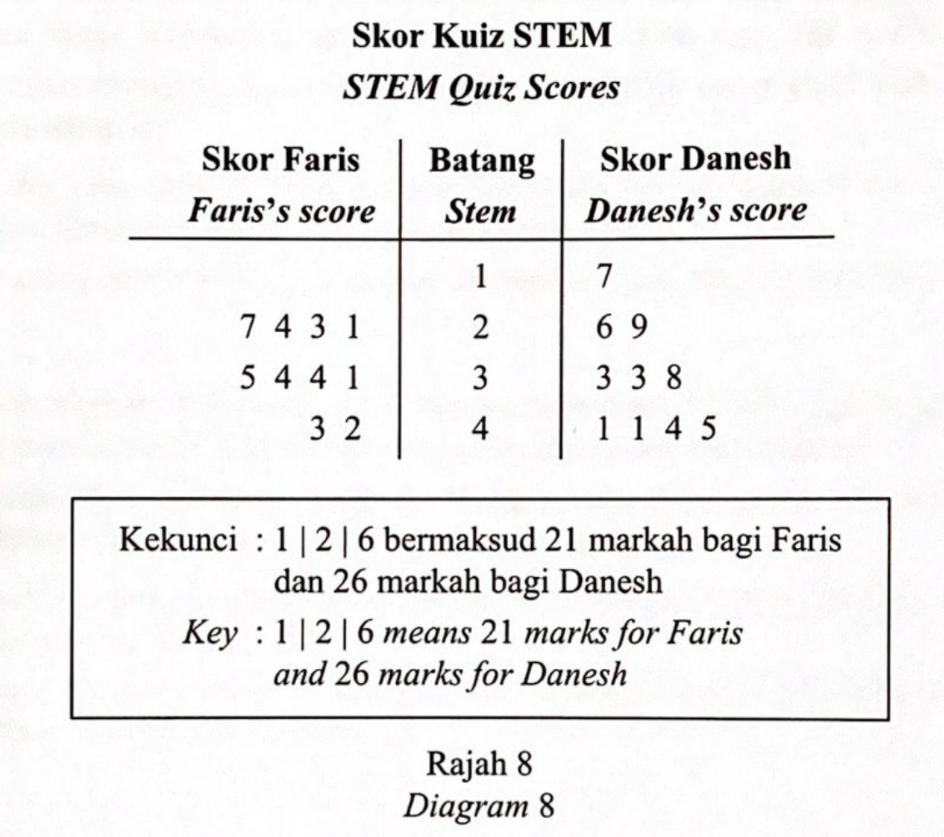
(a) Based on the scores obtained by both participants,
(i) state the mode for each participant, [2 marks]
(ii) calculate the mean score for Faris and Danesh. [2 marks]
(b) Based on the data in Diagram 8, who will be selected for the competition at the national level? Justify your answer. [5 marks]
Solution:
(a)(i)
Mode for Faris = 34
Mode for Danesh = 33 and 41
(a)(ii)
$$ \operatorname{Mean}, \bar{x}=\frac{\sum x}{N} $$
$$ \begin{aligned} &\text { Mean score for Faris }\\ &\begin{aligned} & =\frac{21+23+24+27+31+34+34+35+42+43}{10} \\ & =31.4 \end{aligned} \end{aligned} $$
$$ \begin{aligned} &\text { Mean score for Danesh }\\ &\begin{aligned} & \frac{17+26+29+33+33+38+41+41+44+45}{10} \\ & =34.7 \end{aligned} \end{aligned} $$
(b)
$$ \text { Standard deviation, } \sigma=\sqrt{\frac{\sum x^2}{N}-(\bar{x})^2} $$
$$ \begin{aligned} &\text { Standard deviation for Faris }=\\ &\begin{aligned} & \sqrt{\frac{21^2+23^2+24^2+27^2+31^2+34^2+34^2+35^2+42^2+43^2}{10}-31.4^2} \\ & =7.255 \end{aligned} \end{aligned} $$
$$ \begin{aligned} &\text { Standard deviation for Danesh }=\\ &\begin{aligned} & \sqrt{\frac{17^2+26^2+29^2+33^2+33^2+38^2+41^2+41^2+44^2+45^2}{10}-34.7^2} \\ & =8.427 \end{aligned} \end{aligned} $$
A Selangor state-level STEM quiz competition was conducted to select a representative to the national level. Two participants were involved in the final selection, namely Faris and Danesh. The stem-andleaf plot in Diagram 8 shows the scores obtained by the two participants after several rounds.
(a) Based on the scores obtained by both participants,
(i) state the mode for each participant, [2 marks]
(ii) calculate the mean score for Faris and Danesh. [2 marks]
(b) Based on the data in Diagram 8, who will be selected for the competition at the national level? Justify your answer. [5 marks]
Solution:
(a)(i)
Mode for Faris = 34
Mode for Danesh = 33 and 41
(a)(ii)
$$ \operatorname{Mean}, \bar{x}=\frac{\sum x}{N} $$
$$ \begin{aligned} &\text { Mean score for Faris }\\ &\begin{aligned} & =\frac{21+23+24+27+31+34+34+35+42+43}{10} \\ & =31.4 \end{aligned} \end{aligned} $$
$$ \begin{aligned} &\text { Mean score for Danesh }\\ &\begin{aligned} & \frac{17+26+29+33+33+38+41+41+44+45}{10} \\ & =34.7 \end{aligned} \end{aligned} $$
(b)
$$ \text { Standard deviation, } \sigma=\sqrt{\frac{\sum x^2}{N}-(\bar{x})^2} $$
$$ \begin{aligned} &\text { Standard deviation for Faris }=\\ &\begin{aligned} & \sqrt{\frac{21^2+23^2+24^2+27^2+31^2+34^2+34^2+35^2+42^2+43^2}{10}-31.4^2} \\ & =7.255 \end{aligned} \end{aligned} $$
$$ \begin{aligned} &\text { Standard deviation for Danesh }=\\ &\begin{aligned} & \sqrt{\frac{17^2+26^2+29^2+33^2+33^2+38^2+41^2+41^2+44^2+45^2}{10}-34.7^2} \\ & =8.427 \end{aligned} \end{aligned} $$
Faris will be chosen because the score value is more consistent and the standard deviation value is smaller.