7.2 Measures of Dispersion (Introduction)

7.2 Measures of Dispersion (Introduction)
 
(A) Determine the range of a set of data
1. For an ungrouped data,
Range = largest value – smallest value.

2. For a grouped data,
Range = midpoint of the last class – midpoint of the first class.

Example 1:
Determine the range of the following data.
(a) 720, 840, 610, 980, 900

(b)
Time (minutes)
1 – 6
7 – 12
13 – 18
19 – 24
25 – 30
Frequency
3
5
9
4
4

Solution: 
(a)
Largest value of the data = 980
Smallest value of data = 610
Range = 980 – 610 = 370

(b)
Midpoint of the last class
= ½ (25 + 30) minutes
= 27.5 minute
 
Midpoint of the first class
= ½ (1 + 6) minutes
= 3.5 minute

Range = (27.5 – 3.5) minute = 24 minutes



(B) Medians and Quartiles
1. The first quartile (Q1)is a number such that 1 4 of the total number of data that has a value less than the number.

2. The median is the second quartile which is the value that lies at the centre of the data.
3. The third quartile (Q3) is a number such that 3 4of the total number of data that has a value less than the number.

4. The interquartile range is the difference between the third quartile and the first quartile.

Interquartile range = third quartile – first quartile

Example 2:
 



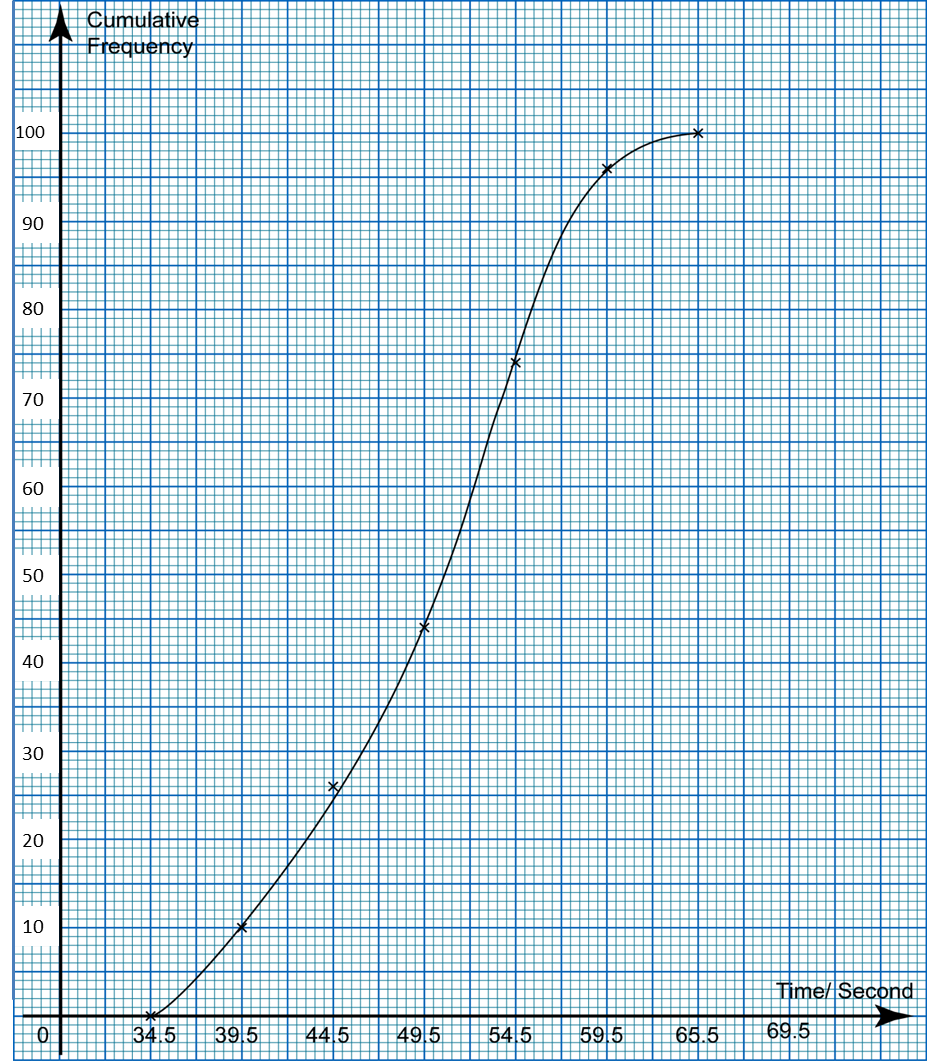
The ogive in the diagram shows the distribution of time (to the nearest second) taken by 100 students in a swimming competition. From the ogive, determine
(a) the median,
(b) the first quartile,
(c) the third quartile
(d) the interquartile range of the time taken.
 
Solution:
 



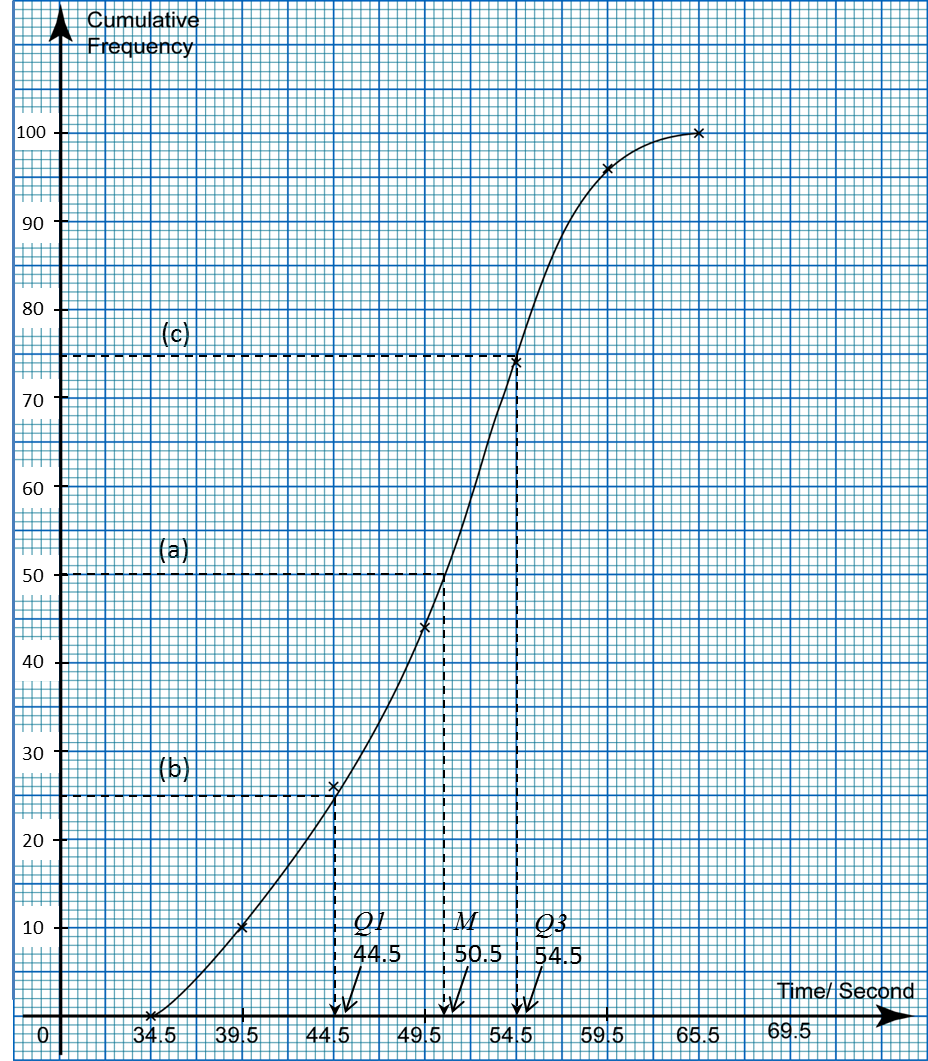
(a) 1 2 of 100 students = 1 2 × 100 = 50 From the ogive, median, M = 50.5 second (b) 1 4 of 100 students = 1 4 × 100 = 25 From the ogive, first quartile, Q 1 = 44.5 second (c) 3 4 of 100 students = 3 4 × 100 = 75 From the ogive, third quartile, Q 3 = 5 4.5 second

(d)
Interquartile range
= Third quartile – First quartile
= 54.5 – 44.5
= 10.0 second 

Leave a Comment