Question 5:
(a) Diagram below shows two points, M and N, on a Cartesian plane.
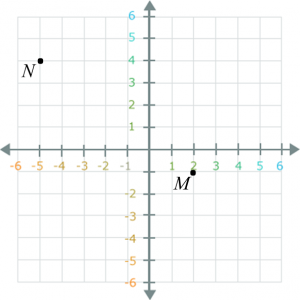
Transformation T is a translation ( 3−1) and transformation R is an anticlockwise rotation of 90o about the centre (0, 2).
(i) State the coordinates of the image of point M under transformation R.
(ii) State the coordinates of the image of point N under the following transformations:
(a) T2,
(b) TR,
(b) Diagram below shows three pentagons, A, B and C, drawn on a Cartesian plane.

(i) C is the image of A under the combined transformation WV.
Describe in full the transformation:
(a) V (b) W
(ii) Given A represents a region of area 12 m2, calculate the area, in m2, of the region represented by C.
Solution:
(a)
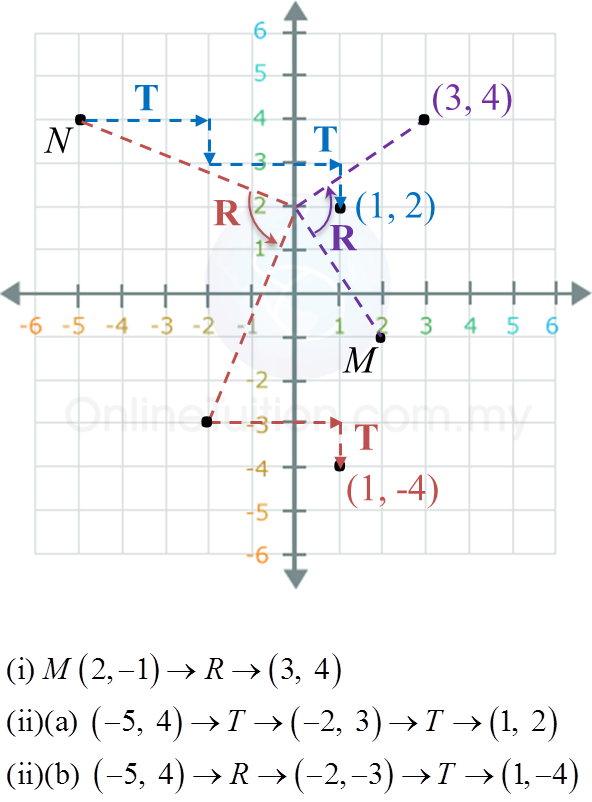
(b)

(b)(i)(a)
V: A reflection in the line x = 8
(b)(i)(b)
W: An enlargement of scale factor 2 with centre (14, 0).
(b)(ii)
Area of B = area of A = 12 m2
Area of C = (Scale factor)2 x Area of object
= 22 x area of B
= 22 x 12
= 48 m2
(a) Diagram below shows two points, M and N, on a Cartesian plane.

Transformation T is a translation ( 3−1) and transformation R is an anticlockwise rotation of 90o about the centre (0, 2).
(i) State the coordinates of the image of point M under transformation R.
(ii) State the coordinates of the image of point N under the following transformations:
(a) T2,
(b) TR,
(b) Diagram below shows three pentagons, A, B and C, drawn on a Cartesian plane.

(i) C is the image of A under the combined transformation WV.
Describe in full the transformation:
(a) V (b) W
(ii) Given A represents a region of area 12 m2, calculate the area, in m2, of the region represented by C.
Solution:
(a)

(b)

(b)(i)(a)
V: A reflection in the line x = 8
(b)(i)(b)
W: An enlargement of scale factor 2 with centre (14, 0).
(b)(ii)
Area of B = area of A = 12 m2
Area of C = (Scale factor)2 x Area of object
= 22 x area of B
= 22 x 12
= 48 m2
Question 6:
(a) Diagram below shows point M marked on a Cartesian plane.
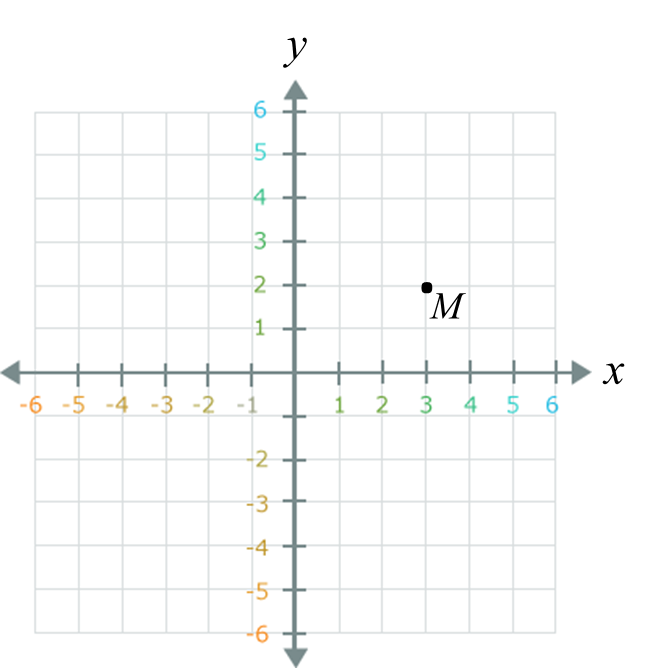
Transformation T is a translation (23) and transformation R is an anticlockwise rotation of 90o about the centre O.
State the coordinates of the image of point M under each of the following transformations:
(i) RT,
(ii) TR,
Solution:
(a)
(b)
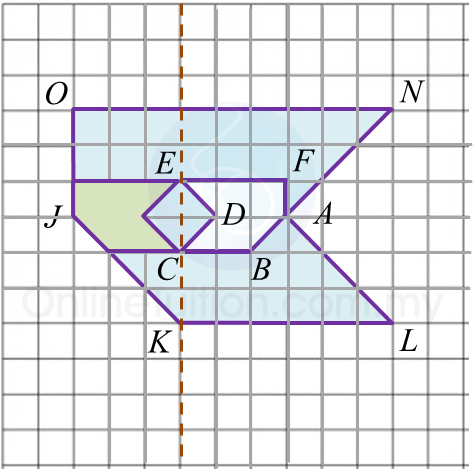
(i)(a)
V: A reflection in the line EC.
(i)(b)
Scale factor=KLBC=62=3W: An enlargement of scale factor 3 at centre J.
(ii)
Area of JKLANO
= 32 x area of ABCDEF
= 9 x 45
= 405 m2
Area of the coloured region
= area of JKLANO – area of ABCDEF
= 405 – 45
= 360 m2
(a) Diagram below shows point M marked on a Cartesian plane.

Transformation T is a translation (23) and transformation R is an anticlockwise rotation of 90o about the centre O.
State the coordinates of the image of point M under each of the following transformations:
(i) RT,
(ii) TR,
(b) Diagram below shows two hexagons, ABCDEF and JKLANO, drawn on square grids.
(i) JKLANO is the image of ABCDEF under the combined transformation VW.
Describe in full the transformation:
(a) W (b) V
(ii) It is given that quadrilateral ABCDEF represents a region of area 45 m2.
Calculate the area, in m2, of the region represented by the shaded region.
Solution:
(a)

(b)

(i)(a)
V: A reflection in the line EC.
(i)(b)
Scale factor=KLBC=62=3W: An enlargement of scale factor 3 at centre J.
(ii)
Area of JKLANO
= 32 x area of ABCDEF
= 9 x 45
= 405 m2
Area of the coloured region
= area of JKLANO – area of ABCDEF
= 405 – 45
= 360 m2