Question 5 (4 marks):
Diagram 5 shows a cylindrical tank in a residential area which have 125 houses. Each house received equal volume of water.
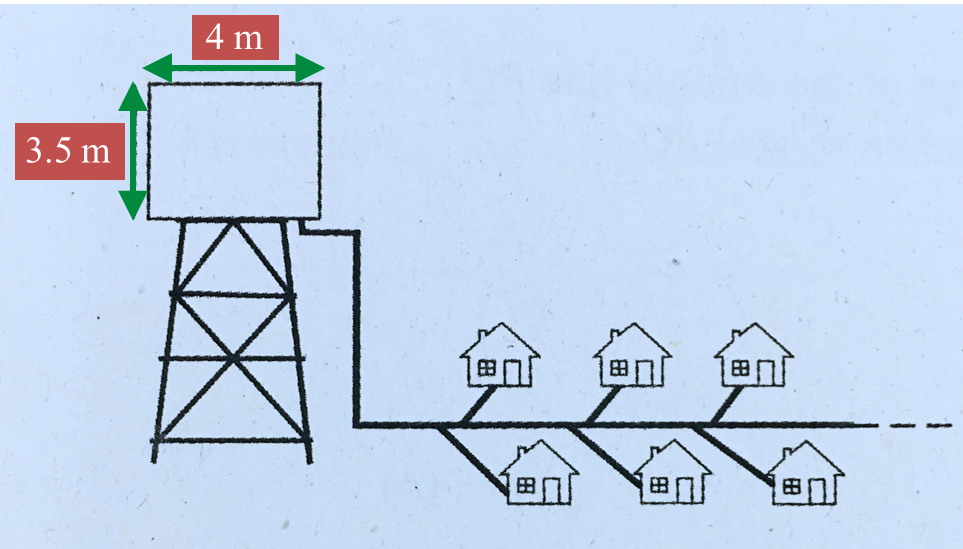 Diagram 5
Diagram 5
The diameter of the cylindrical tank is 4 m. It is given that each house has a cuboid tank with a base area of 0.8 m2.
Using π=227 , calculate the height of the water level, in m, of each tank in the house.
Solution:
Volume of water in cylindrical tank=πr2h=227×22×3.5=44 m3Volume of water in each cuboidtank=441250.8×h=44125h=0.44 m∴Height of water level in each cuboid tank=0.44 m.
Diagram 5 shows a cylindrical tank in a residential area which have 125 houses. Each house received equal volume of water.
 Diagram 5
Diagram 5The diameter of the cylindrical tank is 4 m. It is given that each house has a cuboid tank with a base area of 0.8 m2.
Using π=227 , calculate the height of the water level, in m, of each tank in the house.
Solution:
Volume of water in cylindrical tank=πr2h=227×22×3.5=44 m3Volume of water in each cuboidtank=441250.8×h=44125h=0.44 m∴Height of water level in each cuboid tank=0.44 m.
Question 6 (5 marks):
Diagram 6 shows two parallel straight lines, POQ and RMS drawn on a Cartesian plane.
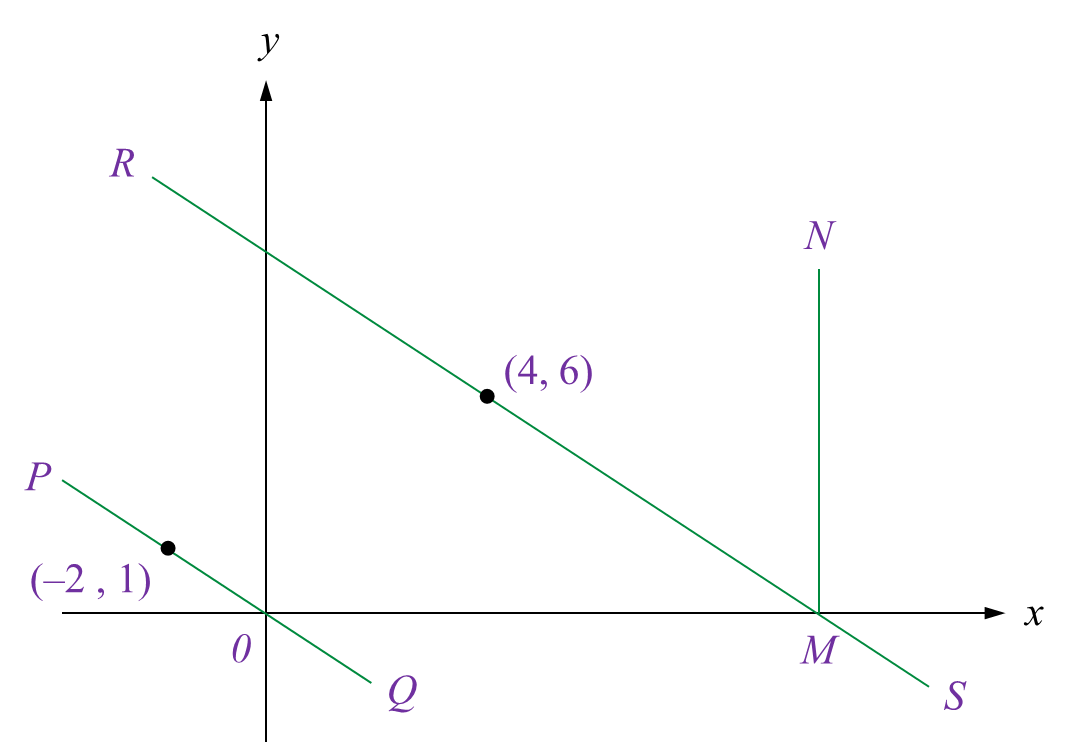 Diagram 6
Diagram 6
Find
(a) the equation of the straight line RMS,
(b) the x-intercept of the straight line MN.
Solution:
(a)
mRMS=mPOQ =1−0−2−0 =−12At point (4, 6)y1=mx1+c6=−12(4)+c6=−2+cc=8The equation of straight line RMS isy=−12x+8
(b)
When y=0,−12x+8=012x=8x=16x-intercept of straight line MN is 16.
Diagram 6 shows two parallel straight lines, POQ and RMS drawn on a Cartesian plane.
 Diagram 6
Diagram 6Find
(a) the equation of the straight line RMS,
(b) the x-intercept of the straight line MN.
Solution:
(a)
mRMS=mPOQ =1−0−2−0 =−12At point (4, 6)y1=mx1+c6=−12(4)+c6=−2+cc=8The equation of straight line RMS isy=−12x+8
(b)
When y=0,−12x+8=012x=8x=16x-intercept of straight line MN is 16.